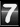Day: March 31, 2017

SU(1,1) parametrization

Posted on by arkajad

In the last post, while getting hyperbolic, we have met the Lie group SU(1,1) – the group of 2\times 2 complex matrices A of determinant one, \det A=1, and such that

(1)   \begin{equation*} AGA^*=G,\end{equation*}

where

(2)   \begin{equation*}G=\begin{bmatrix}1&0\\0&-1\end{bmatrix},\end{equation*}

and star A^* is Hermitian conjugated matrix to A.

It was our first meeting here with SU(1,1), and there was no enough time to decide whether we want to make friends with this group or not. Today we will look at SU(1,1) closer, in particular at her shape. I think we will like her … She has, as we will see below, the shape of the inside of a solid torus, donut-like shape:

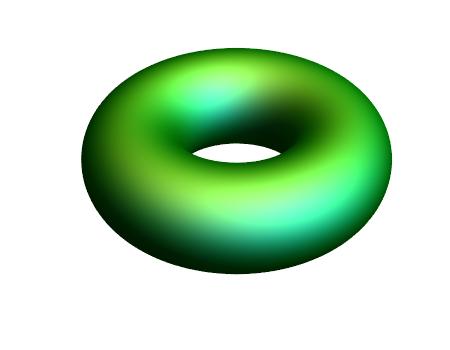
How do we get this donut? One method is to visit a backery, another method is by doing some little algebra. We will choose the second method. In fact, we are going to get not only the shape of the group itself, but also the shape of the disk that the group is acting on! It will be the cross-section of the torus:

Let us take a matrix A from SU(1,1). It is a complex 2\times 2 matrix, so let us write it as

(3)   \begin{equation*}A=\begin{bmatrix}\lambda&\mu\\ \nu&\rho\end{bmatrix}.\end{equation*}

From \det A=1 we get

(4)   \begin{equation*}\lambda\rho-\mu\nu=1.\end{equation*}

On the other hand we have

(5)   \begin{equation*}AGA^*=\begin{bmatrix}\lambda&\mu\\ \nu&\rho\end{bmatrix}\begin{bmatrix}1&0\\0&-1\end{bmatrix}\begin{bmatrix}\bar{\lambda}&\bar{\nu}\\ \bar{\mu}&\bar{\rho}\end{bmatrix}=\begin{bmatrix}\lambda\bar{\lambda}-\mu\bar{\mu}&\lambda\bar{\nu}-\mu\bar{\rho}\\\nu\bar{\lambda}-\rho\bar{\mu}&\nu\bar{\nu}-\rho\bar{\rho}\end{bmatrix},\end{equation*}

and so from the determining Eq. (1) we must have

(i)   \begin{align*} |\lambda|^2-|\mu|^2&=1,\\ |\rho|^2-|\nu|^2&=1,\tag{ii}\\ \lambda\bar{\nu}-\mu\bar{\rho}&=0,\tag{iii}\\ \nu\bar{\lambda}-\rho\bar{\mu}&=0.\tag{iv} \end{align*}

Eq. (iv) is just a complex conjugation of (iii), so it does not bring us any new information. The whole information is contained in the first three equations.

From the first two equations we deduce that, since |\lambda|^2=1+|\mu|^2\geq 1 and |\rho|^2=1+|\nu|^2\geq 1, therefore \lambda and \rho are non-zero. From Eq. (iii), dividing by non-zero \bar{\rho}, we get

(6)   \begin{equation*} \mu=\frac{\lambda\bar{\nu}}{\bar{\rho}},\end{equation*}

which we substitute into Eq. (4)

    \[\lambda\rho-\frac{\lambda|\nu|^2}{\bar{\rho}}=1,\]

or, multiplying both sides with \bar{\rho},

    \[\lambda|\rho|^2-\lambda|\nu|^2=\bar{\rho}.\]

Then, using Eq. (ii), we get \lambda=\bar{\rho}, thus

(7)   \begin{equation*}\rho=\bar{\lambda}.\end{equation*}

Therefore, from Eq. (6), we get \mu=\bar{\nu}, or

(8)   \begin{equation*}\nu=\bar{\mu}.\end{equation*}

So, we obtain that every matrix A of SU(1,1) is of the form

(9)   \begin{equation*}A=\begin{bmatrix}\lambda&\mu\\ \bar{\mu}&\bar{\lambda}\end{bmatrix}\end{equation*}

where

(10)   \begin{equation*}|\lambda|^2-|\mu|^2=1.\end{equation*}

Conversely, every matrix of this form is in SU(1,1).

Now, we will determine the shape of our beauty.

Since |\lambda|\geq 1, we can write

(11)   \begin{equation*} \lambda=|\lambda|e^{i\theta},\end{equation*}

where \theta is the uniquely defined phase, 0\leq\theta<2\pi.

Let

(12)   \begin{equation*} z=\frac{\bar{\mu}}{\lambda}.\end{equation*}

Then, using Eq. (10)

(13)   \begin{equation*} |z|^2=\frac{|\mu|^2}{|\lambda|^2}=\frac{|\lambda|^2-1}{|\lambda|^2}=1-\frac{1}{|\lambda|^2}<1.\end{equation*}

Thus z is in the interior of the unit disk in the complex plane.

Conversely, given \theta and z, with |z|<1 we can determine uniquely \lambda and \mu. From Eq. (13) we set

(14)   \begin{equation*}|\lambda|=\frac{1}{\sqrt{1-|z|^2}},\end{equation*}

then
Finally, using Eq. (12), we set

(15)   \begin{equation*}\mu=\bar{\lambda} \bar{z}.\end{equation*}

Summarizing, the shape of SU(1,1) is that of the Cartesian product of the circle parametrized by \theta, 0\leq\theta<2\pi, and of the interior of the unit disk, parametrized by complex numbers z with |z|<1. That is the interior of the solid torus, that is the donut without its skin.

Pretty and tasty. Not necessarily healthy, though….