Hyperbolic geometry – Playing with parallels

Posted on 2017/04/07 by arkajad

In SU(1,1) straight lines on the disk we have seen nice families of parallel straight lines in the hyperbolic geometry of the unit disk of the complex plane. Here is one such family:

Family of parallel straight lines

Let us recall how was it obtained. We start with a template straight line through the origin o at z=0..

Template straight line

Then we take one-parameter group of SU(1,1) transformations A_1(t) generated by X_1

(1)   \begin{equation*}X_1=\begin{bmatrix}0&i\\-i&0\end{bmatrix},\end{equation*}

(2)   \begin{equation*}A_1(t)= \exp(tX_1)=\begin{bmatrix}\cosh(t)&i\sinh(t)\\-i\sinh(t)&\cosh(t)\end{bmatrix}.\end{equation*}

We apply transformations A_1(t) to our template straight line (we use fractional linear transformations explained in previous posts), for several different values of t, and we obtain the family depicted above.

Let us take a particular case, with t=-0.4.

We have \sinh(-0.4)=-0.410752, \cosh(-0.4)=1.08107, therefore the point (x,0) on the template line is transformed into p(x) given by

(3)   \begin{equation*}p(x)=\frac{1.08107 x+0.410752 i}{1.08107-0.410752 x i}.\end{equation*}

The whole template line is transformed as follows

Template and one parallel

We obtain another straight line, parallel to the original one (as the two lines do not intersect). If we rotate this new line clockwise about the origin, at a certain point the two lines touch at z=1. That is the limit of being parallel. If we rotate further, then the two lines start to intersect.

Let us calculate the angle \phi that we need to use to reach the limit. We set x=1 in Eq. (3) and solve for \phi such that

(4)   \begin{equation*}e^{i\phi}=\frac{1.08107+0.410752 i}{1.08107-0.410752 i}.\end{equation*}

Solving this I am finding (in radians)

(5)   \begin{equation*}\phi=0.726204822741529.\end{equation*}

Our U(1) rotation group is defined by matrices

(6)   \begin{equation*}U(t)=\begin{bmatrix}e^{it}&0\\ 0&e^{-it}\end{bmatrix}.\end{equation*}

It acts on complex numbers by:

(7)   \begin{equation*}U(t):z\mapsto e^{-2it}z.\end{equation*}

Therefore in order to obtain the limiting line we need to apply U(\phi/2)

(8)   \begin{equation*}U(\phi/2)=\begin{bmatrix} 0.934799+0.355176 i & 0 \\ 0 & 0.934799-0.355176 i \end{bmatrix}\end{equation*}

The total transformation U(\phi/2)P(-0.4) that we will use is therefore

(9)   \begin{equation*}\begin{bmatrix} 1.01059\, +0.383971 i & 0.145889\, -0.383971 i \\ 0.145889\, +0.383971 i & 1.01059\, -0.383971 i \\ \end{bmatrix}.\end{equation*}

Acting on the point x on the real axis it produces a point p(x) in the disk

(10)   \begin{equation*}p(x)=\frac{(1.01059\, -0.383971 i) x+(0.145889\, +0.383971 i)}{(0.145889\, -0.383971 i) x+(1.01059\, +0.383971 i)}\end{equation*}

    \[=\frac{(1.7477 + 4.43167 x + 1.7477 x^2)+(1.9679 - 3.93579 x + 1.9679 x^2)i}{x^2+6.92707}.\]

The real part, 1.7477 + 4.43167 x + 1.7477 x^2 vanishes for x=-0.488459, The imaginary part has, for this x the value 0.608442. That means that at z=0.608442 i the limiting line crosses the vertical imaginary axis.

Template and the limiting parallel

We could easily repeat the above reasoning applied, this time, to the left point z=-1. We would get the second limiting line. The two lines cross at z_0=0.608442 i, as shown below:

Template and two limiting parallels

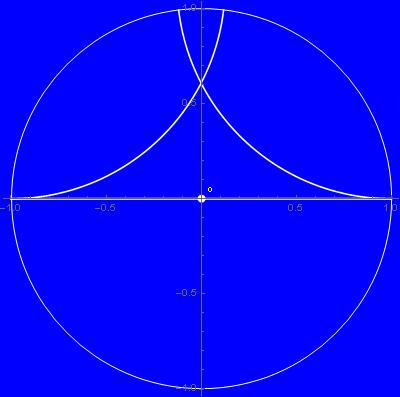
We will continue our adventure in the next post. We will produce there this image of parallel lines – the Angel of Geometry

Template and parallels through one common point.

Print 🖨 PDF 📄 eBook 📱
This entry was posted in Geometry, Hyperbolic geometry, SU(1,1). Bookmark the permalink.

12 Responses to Hyperbolic geometry – Playing with parallels

  1. Bjab says:
    2017/04/07 at 23:28

    Arku,
    należy mi się wyjaśnienie jaka jest przyczyna tego, że w niniejszym poście wzór (2) wygląda jak wygląda.

    Mam kilka hipotez.

    1. Wzór ten edytowałeś dzisiaj od początku ze swojej biologicznej pamięci. (mało prawdopodobne)

    2. Wzór ten edytowałeś dzisiaj od początku na podstawie swoich błędnych odręcznych notatek, których nie poprawiłeś. (mało prawdopodobne)

    3. Wzór ten skopiowałeś dzisiaj z jakiś swoich niepublikowanych cyfrowych zasobów, w których nie naniosłeś poprawki. (uporczywe istnienie i powielanie błędu – podobnie jak z kodem DNA)

    4. Niniejszy post dzisiaj opublikowany napisałeś wcześniej niż dwa dni temu.

    • arkajad says:
      2017/04/09 at 14:02

      My answer was incomplete. Writing a post I have to compile latex formulas many-many times, to see if they are correct. I am often making latex errors. Writing online, each time I compile, an external latex server must be connected. So, first I write everything as a latex file and compile on my PC. So, for each post I have a a latex draft on PC. The draft is not always exactly the same as the post, since latex on PC would not compile with some tags that are being used by the blogging software.

      • Bjab says:
        2017/04/13 at 11:00

        “The draft is not always exactly the same as the post”
        So it is rather version 3) not 2).

        Taking care of databases consistency is a painful job, but necessary.

        • arkajad says:
          2017/04/13 at 11:34

          Yes, indeed. I am learning. Probably it would be useful to have all important and true formulas collected in one document, but there is not enough time to do that …

  3. Bjab says:
    2017/04/09 at 08:03

    Should there be minus in exponent in (7) ?
    (with consequences)

Leave a Reply