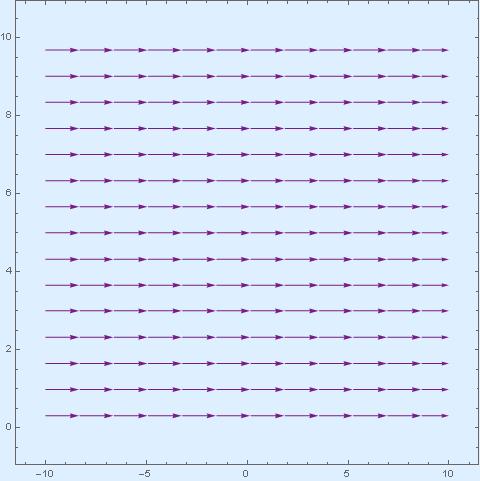
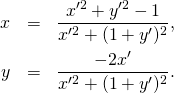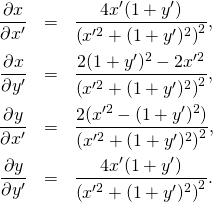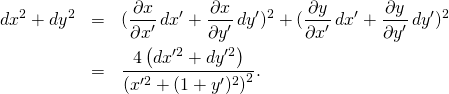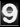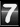In the recent note “SL(2,R) generators and vector fields on the half-plane” we got acquainted with two particular simple symmetries of the non-Euclidean hyperbolic geometry of the complex upper half-plane ![]() These two symmetries are horizontal translation and uniform dilation. The horizontal translational symmetry means that all geometric properties of objects are invariant when all parts of the object keep the same height (constant
These two symmetries are horizontal translation and uniform dilation. The horizontal translational symmetry means that all geometric properties of objects are invariant when all parts of the object keep the same height (constant ![]() ), and only the
), and only the ![]() coordinate is translated by a fixed amount. The symmetry with respect dilations means that when we multiply all
coordinate is translated by a fixed amount. The symmetry with respect dilations means that when we multiply all ![]() coordinates by a certain positive number, then we should also multiply the
coordinates by a certain positive number, then we should also multiply the ![]() coordinates by the same number. We obtain this way not only an object that is similar to the original, but, moreover, it also has the same size!
coordinates by the same number. We obtain this way not only an object that is similar to the original, but, moreover, it also has the same size!
In Hyperbolic angels and demonswe were playing with “equal sizes” of Esher’s angels and demons on the Poincare disk:

Today we will use the Cayley transform and move the Esher’s art piece to the upper half-plane.

The exact name of the image we will be playing with is Circle Limit IV. We want to render this image on the upper half-plane using Cayley transform. The problem that we need to address is this: to render the whole circle, we would need the whole upper half-plane. But we can only show a finite part of the plane. Therefore we need to decide how to cut?
I created a little Mathematica program to help me with this decision:

I decided to use ![]() and
and ![]() The part of the disk that is not being used is then small enough not to worry about (on the right in the image, near
The part of the disk that is not being used is then small enough not to worry about (on the right in the image, near ![]() on the circle boundary ). That gives the ratio 3:2 of the image. I decided to create 1440×960 pixels image. For this I had to find on the net Esher’s Limit Circle with high enough resolution. I have found it here. I cropped it to remove border and ended up with 2272×2276 image. In principle it should be a square, but few pixels should not make a difference. So, here is my Mathematica code: importing the image, converting to table, converting pixels to coordinates, making Cayley transform to the disk, converting disk coordinates to pixels, reading the color, using it for coloring the pixel on the half-plane;
on the circle boundary ). That gives the ratio 3:2 of the image. I decided to create 1440×960 pixels image. For this I had to find on the net Esher’s Limit Circle with high enough resolution. I have found it here. I cropped it to remove border and ended up with 2272×2276 image. In principle it should be a square, but few pixels should not make a difference. So, here is my Mathematica code: importing the image, converting to table, converting pixels to coordinates, making Cayley transform to the disk, converting disk coordinates to pixels, reading the color, using it for coloring the pixel on the half-plane;

And here is the end result:
All these angels have the same size, when measured with the hyperbolic stick, that was possibly created by the hyperbolic devils, that are all of the same size as well.

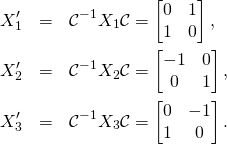
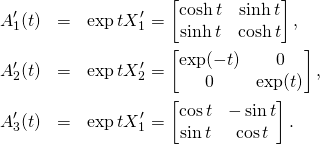
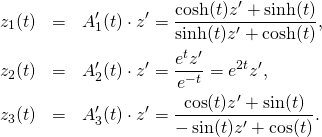

 on the half-plane
on the half-plane 
 on the half-plane
on the half-plane 
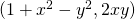 on the half-plane
on the half-plane 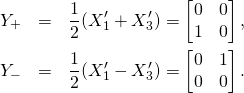

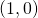 on the half-plane
on the half-plane