Category: Moments of inertia

1, 2, 3 and butterfly effect

Posted on by arkajad

I know: my blog is somewhat chaotic. But what is chaos? From “Celestial Mechanics: The Waltz of the Planets” by Alessandra Celletti and Ettore Perozzi:


Chaos From the Greek Χάος, denoting primordial emptiness. The term is used to indicate the extreme sensitivity of a trajectory to the initial conditions, which implies unpredictable dynamical behaviour in the corresponding dynamical system.

With T-handle floating in space and flipping for no apparent reason – it looks like we are in a chaotic mode:

But are we? Where is “the extreme sensitivity of a trajectory to the initial conditions“? We have to address this question. The waltz of planets can be, in some respect, chaotic. But sometimes it is well organized. Like this waltz:

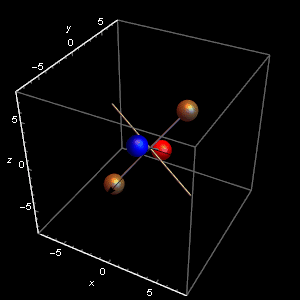
1,2,3 and 1,2,3, and my T-handle that replicates the cosmic behavior in the Dzhanibekov effect (see my previous posts) has principal moments of inertia 1,2,3. Why? What 1,2,3 has to do with chaos?

Time to explain. In general, when moons and planet spin, their motion is not chaotic. But sometimes it can be. Let us take my T-handle with principal moments of inertia I_1=1,I_2=2,I_3=3 (see my previous post).

Spin it about its shorter axis. It may show the strange flipping behavior – see the animated gif below (click to open it)

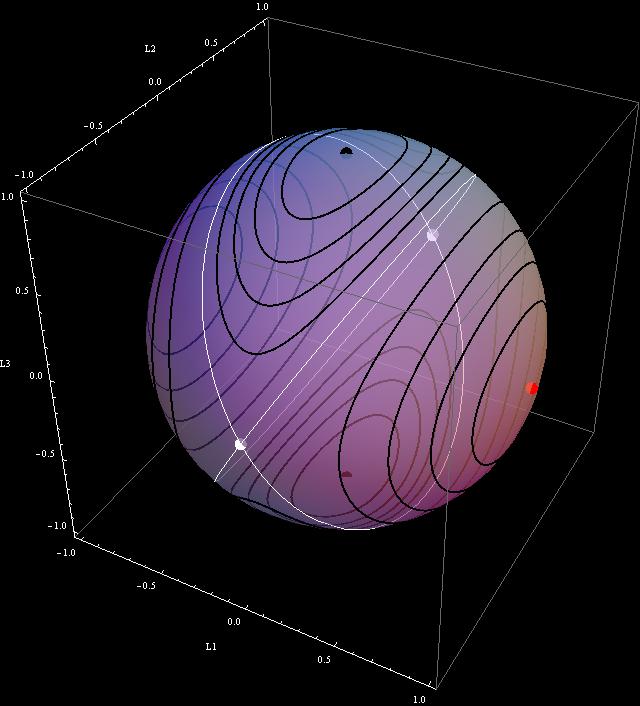
The “extreme sensitivity of a trajectory to the initial conditions” happens when the parameter d, that is the ratio of the doubled kinetic energy to squared angular momentum vector, is close to 1/I_2. The motion is governed by Jacobi elliptic functions \cn,\sn,\dn. The period \tau of these functions directly affects the time spent by the T-handle between flips. And this period is a very sensitive function of d, when d is close to 1/I_2.
Here is the graph of \tau for our T-handle:

The formula for \tau(d) involves elliptic K function. But for d very close to 1/I_2 there is an approximate (asymptotic) formula derived in the paper “Janibekov’s effect and the laws of mechanics” by A.G. Petrov and S. E. Volodin, Doklady Physics}, 58(8):349–353, 2013:

    \[\tau_0(d)=2\log\frac{16}{|\mu|},\]

where

    \[\mu=\frac{I_2(1-d I_2)(I_3-I_1)}{(I_3-I_2)(I_2-I_1)}.\]

Here is how the two formulas compare:

The blue curve is the simple asymptotic formula.

But why 1,2,3?

Why? Because 1,2,3 is optimal. But what is optimal?

How do we know if 9 to 5 is the most optimal time to work? – Quora
We don’t and we never will know, because everyone is different.

So, what did I optimize to get my 1,2,3? First I had a vague feeling that this is good

Genesis 1:31 God saw all that he had made, and it was very good …

But then I was able to prove that it was good by optimizing \tau(d). The dimensionless parameter \epsilon=1-d I_2 tells us how close we are to the critical value of d=1/I_2. Being very close, near the peak in the graphs above, we want to know what should be the values of I_1,I_2,I_3 for which the period \tau is maximal? We use the approximate formula above. To find where is the maximum of \tau, with \epsilon fixed, is to find where is the minimum of the ratio:

    \[\frac{I_2(I_3-I_1)}{(I_3-I_2)(I_2-I_1)}.\]

Here I_1,I_2,I_3 cannot be completely arbitrary. The moments of inertia should satisfy the inequality I_1+I_2\geq I_3. Suppose I_2=2. What should be I_1,I_2? I used Mathematica to get the answer:

If I assume that I_2=2, then I_1=1,I_3=3. If I assume that I_2=20, then I_1=10,I_3=30. Simple?

So, even if everyone is different, even if there is no optimal answer that would satisfy everybody, there is an optimal answer to my needs, and that is 1,2,3.

“In trying to please all, he had pleased none.”
― Aesop, Aesop’s Fables

With 1,2,3 we have more time to see the butterfly flying. By flipping its wings it can change dramatically the period of the flying T-handle on the other side of the planet.

Dzhanibekov effect – handling the T-handle

Posted on by arkajad

Errors that you remember most are the errors you have made yourself. Toys that you remember most are the toys that you have made yourself. As a kid I was making all kind of toys. Planes that would fly. Slings that would shoot. But also this funny toy that I was not able to find on Youtube in English. I found it only in Polish. Here it is – the “worm”:

And here are more detailed instructions with narration in Polish, but it should not matter, because you see all the details:

The bearing ball inside, invisible, makes it to behave strangely, the “worm” flips and jumps, almost like being alive.
So much about kid’s toys. Now we are playing with another toy, that also looks like being alive. Except that it whirls and flips in space, on ISS space station:

Dzhanibekov effect is the name. It does not have a bearing ball inside. What is hidden inside are Jacobi elliptic functions. They make it to flip, for no apparent reason at all, and do it, as it seems, periodically. Why? Dzhanibekov, the Russian cosmonaut who has discovered the effect, didn’t know why. He wrote to me that it is still a puzzle for him, though he prefers now to concentrate on “quantum puzzles”.
The movie above shows a T-handle. So, in today’s post I will construct my own T-handle that will whirl and flip the same way, but without going to space. We will do it with graphics, with math, with numbers.

I want to build a T-handle with principal moments of inertia 1,2,3 (I like this sequence). It will look like this:

But I want to make it as simple as possible, so I will make it very-very thin. Mathematically: I will make it of lines rather than tubes:

One line is along the x-axes. I fix it to have length L_1=2, from x=-1, to +1. For the metal I choose the linear density to be \mu=3. So the mass of the horizontal tube is 6. The length of the vertical part is to be found. I want it to be such that the principal moments of inertia with respect to the center of mass are 1,2,3.

First we need to find the formula for the center of mass position y. It is clear, from symmetry, that the center of mass will have x-coordinate zero. We need a formula for y. The center of mass of the horizontal part, that has mass m_1=6, is at (0,0). The center of mass of the vertical part, which has mass 3L_2, is at (0,L_2/2). Thus the y coordinate of the center of mass of our T-handle is

    \[y=\frac{m_1\times 0 + m_2\times L_2/2}{m_1+m_2}=\frac{3L_2^2/2}{6+3L_2}= \frac{L_2^2}{4+2L_2}.\]

Now we need to calculate the moments of inertia. First the moment of inertia with respect to the y-axis.
Wikipedia has a List of moments of inertia. There we find that for a cylinder of length L and mass m the moment of inertia with respect to the axis through its center is mL^2/12. I checked, calculating it myself, and it is correct. Here is how you can calculate it:

    \[I=2\int_0^{L/2}x^2\mu dx=2\mu\frac{x^3}{3}|_{L/2}=2\mu\frac{L^3}{3\times 8}=m\frac{L^2}{12}.\]

In our case only L_1 contributes to the moment of inertia with respect to the y-axis, because we assume our cylinders to be of zero diameter. And the contribution from L_1 is 6\frac{2^2}{12}=2. This is our I_2:

    \[I_2=2.\]

Our task is now to choose the Length L_2 so that the moment of inertia I_1 of the whole body with respect to the horizontal axis through y is 1.
It will be convenient to use what is called “Parallel axis theorem“. From Wikipedia:

First consider the contribution from L_1. The mass is m_1=6, the distance is d=y, so the contribution is

    \[I_{1,1}=0+6y^2.\]

The first term here is 0 since the moment of inertia of L_1 with respect to the x-axis is zero.
Then the contribution from L_2:

    \[I_{1,2}=\mu L_2 \frac{L_2^2}{12}+\mu L_2(L_2/2-y)^2.\]

Substituting the expression for y, after some little calculation, we get

    \[I_1=I_{1,1}+\[I_{1,2}=\frac{L_2^3(8+L_2)}{4(2+L_2)}.\]

We now solve the equation L_2=1. This is fourth degree equation, we find that two roots are real, and only one is positive:

    \[L_2=1.10938.\]

This is our T-handle.
And here is the animation – with Jacobi elliptic functions inside. The T-handle is flipping, as if being alive, with elliptic functions inside:

Click on the image to open the gif animation.

The Flipping Top Movie that they will not show you in the movie theaters!

Posted on by arkajad

In the last post, Racket about tennis racket, I did not quite finish with the critical appraisal of the Poinsot-type picture:

According to wikiHow to Critique Artwork I should really do the following:

2
Analyze the artwork. Evolve the art criticism from a technical description to an in-depth examination of how the technical elements were utilized by the artist to create the overall impression conveyed by the artwork. Technical elements you need to analyze when you critique artwork include:

Color.
Shapes, forms and lines.
Texture.
Light and shadow.
How each technical element contributes to the mood, meaning and aesthetic sensation of the artwork.

But even before doing that, there is something very very very important that was in part 1: “Objects in the painting” – that I did not delve deep enough into. The point is that the artist is presenting here certain particular impressions about a certain object. And the object is not some abstract sphere in some “angular momentum space”. The object is real, is pretty, is simple, and is mysterious – in our ordinary 3D space, floating over the water, under blue cloudy skies. Here it is:

The Top object

So, what is this object? It is not a drone. More like an UFO. It consists of four spherical masses. It is not important how large these spheres are. Here they seem to be quite large, but, in fact, life is simpler when they shrink to points. What is important is that they are heavy. Each mass in the picture is, say, 1/2 kg. The blue and red masses are connected by a weightless rod of length 2m. The bronze metal masses are connected by a weightless rod that is 2*\sqrt{2}\approx 2.83 m long. There is also the third, thin and weightless, rod that is perpendicular to the other two. It plays no role whatsoever, no masses are attached to its ends. It is there just for pure pleasure of the Creator.

The center of mass of our top is at its geometrical center, where the joining rods intersect. Let us agree that the axis joining the bronze masses is the first, say, x-axis. The axis joining red and blue masses is the second, y-axis, and the perpendicular axis, with no masses attached, is the third, the z-axis.

Let’s recall how we calculate the moments of inertia of a system of masses. Here is the extract from Hyperphysics site:

We calculate now the moment of inertia I_ with respect to the first axis. There are two masses, each m=1/2, rotating about this axis, each at the distance 1. The moment of inertia (I will skip the units, like kg and m) is

    \[ I_1=\frac{1}{2}\, 1^2+\frac{1}{2}\,1^2=1.\]

Now we calculate I_2

    \[I_2= \frac{1}{2}\,(\sqrt{2})^2+\frac{1}{2}\,(\sqrt{2})^2=2.\]

And I_3

    \[I_3= =\frac{1}{2}\, 1^2+\frac{1}{2}\,1^2+\frac{1}{2}\,(\sqrt{2})^2+\frac{1}{2}\,(\sqrt{2})^2=3.\]

Now let us see these moments of inertia in a spectacular action of freely rotating in space. Not just rotating! Rotating and the flipping.

Click on the image to open the animation in a new window. Depending on your connection it may take a while. The size is almost 1 MB. You will see the flips. But why? What causes these flips?

Yes, these balls do move and flip! As if they were alive! Yes, rotating and spinning and flipping opens the door of perception to other dimensions. And we will learn it all. No joking, it all. But now we have a movie, and we will have to learn about How to Write a Movie Review…