Abstract: Contrary to the claim by Alexey Nikulov it is argued that there is no error in Landau and Lifshitz derivation of magnetic coupling formula (113.2)
In [1], Sec. 4.3, “Quantum mechanics cannot describe both opposite cases”, Alexey Nikulov claims that Landau and Lifshitz have made an error deriving, in their quantum theory textbook [2], the formula for the coupling of the angular momentum to the magnetic field in their chapter on “An atom in a magnetic field”. More specifically Nikulov claims in [1] that the formula (113.2) in [2] is obtained by Landau and Lifshitz from (113.1) by an “illegal substitution” or, by an “elementary arithmetic error” [3].
While it is true that Landau and Lifshitz could have been more explicit in their derivation, I will derive their (113.2) from (113.1) in all detail – showing that their is no “arithmetic error”, and that it is, in fact, Alexey Nikulov who made an error misunderstanding the arguments in Ref.[2] and “overshooting” with his criticism.
As it is very important to pay attention to details, let us start with an exact image (see Fig. 1) of the starting formula (113.1) in Ref. [2]:
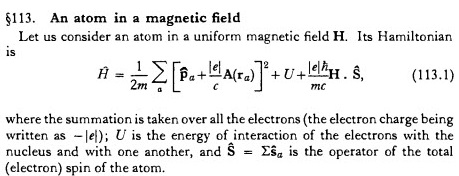
Fig. 1. Landau-Lifshitz p.461, Eq. (113.1)
A. Nikulov is questioning the derivation, given in Ref. [2], of the following formula copied here from Ref. [2] in Fig. 2 below.

Fig. 2, Landau-Lifshitz p.461, Eq. (113.2)
The Bohr magneto ![]() is defined here as
is defined here as ![]() We will neglect spin
We will neglect spin ![]() and the scalar potential
and the scalar potential ![]() , and write down the the starting formula just for one electron, setting
, and write down the the starting formula just for one electron, setting ![]() :
:
Remark: These simplifying assumptions can be made without losing the essence of the argument.
Thus our starting formula, which we denote as ((113.1)) reads as follows
((113.1)) ![]()
(1) ![]()
is the canonical momentum vector operator and ![]() is the vector potential for the magnetic field
is the vector potential for the magnetic field ![]() :
:
(2) ![]()
The electron charge (negative) is ![]() .
.
In order to derive the formula (113.2) of Ref. [2] we need to calculate the Hamiltonian ![]() explicitly. To this end we take the square:
explicitly. To this end we take the square:
(3) 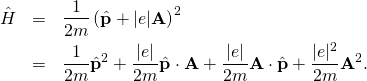
Following Ref. [2] we introduce ![]() defined as
defined as
(4) ![]()
where ![]() is the canonical momentum defined in Eq. (1).
is the canonical momentum defined in Eq. (1).
Then, using Eq. (4) we can rewrite (3) as
(5) ![]()
The vector potential ![]() is a function of coordinates, therefore in general it will not commute with the components of the canonical momentum operator. We have
is a function of coordinates, therefore in general it will not commute with the components of the canonical momentum operator. We have
(6) ![]()
We consider the case of a uniform magnetic field, i.e. the vector ![]() is constant. Then
is constant. Then ![]() can be chosen as
can be chosen as
((111.7)) ![]()
In this case, with ![]() given by ((111.7)) we have
given by ((111.7)) we have ![]()
therefore ![]() . Thus
. Thus ![]() given by Eq. (3), takes the form:
given by Eq. (3), takes the form:
(7) ![]()
or, making use of Eq. ((111.7)) again:
(8) ![]()
Using the standard vector product identity
(9) ![]()
we can rewrite the second term to obtain
(10) ![]()
We now introduce canonical angular momentum operator ![]() defined as
defined as
(11) ![]()
Then
(12) ![]()
The above is Eq. (113.2) of Ref. \cite{LandauL} that we have derived step by step, and without any “arithmetic errors”. Therefore the part of Ref. \cite{nikulov2016} questioning the validity of this derivation is erroneous.
It is nevertheless interesting to try to understand why such an wrong conclusion could arise. The following is my guess based on the following sentence in Sec. 4.3 of Ref. [1]:
\begin{quotation}“The additional summand ![]() could appear in the relation (113.2) due to illegal substitution of
could appear in the relation (113.2) due to illegal substitution of ![]() by
by ![]() in
in ![]() ”\end{quotation}
”\end{quotation}
While in quantum mechanics we do have the canonical momentum operator (see Eq. (1), there is no canonical velocity operator. The explicit expression for the velocity operator must be calculated for each Hamiltonian separately. Given a Hamiltonian ![]() (assuming not time dependent) the corresponding velocity operator is defined by
(assuming not time dependent) the corresponding velocity operator is defined by
(13) ![]()
Its expectation value ![]() is equal to the time derivative of the expectation value of the position operator.
is equal to the time derivative of the expectation value of the position operator.
![]() we find that
we find that
the expression for the velocity operators is given by
(14) ![]()
For a particle in a magnetic field ![]() the Hamiltonian
the Hamiltonian ![]() is defined by Eq. ((113.1)), that is:
is defined by Eq. ((113.1)), that is:
(15) ![]()
where the kinetic momentum ![]() is defined as
is defined as
(16) ![]()
In this case the velocity operator ![]() is given by a different expression than that in the free case:
is given by a different expression than that in the free case:
(17) ![]()
In both cases the Hamiltonian can be written as the kinetic energy ![]() but the expression in terms of the canonical momenta and positions is different in each case. Nikulov seems to claim that it is impossible to derive the second term in Eq. ((113.2)), the one containing the coupling of the magnetic field to the angular momentum, from “just the kinetic energy” because he fails to notice that the kinetic energy’ has a different expression for a particle in a magnetic field than the one without. It is for this reason that the whole section of Ref. [1] needs to be completely rewritten.
but the expression in terms of the canonical momenta and positions is different in each case. Nikulov seems to claim that it is impossible to derive the second term in Eq. ((113.2)), the one containing the coupling of the magnetic field to the angular momentum, from “just the kinetic energy” because he fails to notice that the kinetic energy’ has a different expression for a particle in a magnetic field than the one without. It is for this reason that the whole section of Ref. [1] needs to be completely rewritten.
References
[1] Alexey Nikulov, Could ordinary quantum mechanics be just fine for all practical purposes?, Quantum Stud.: Math. Found. (2016) 3: 41., doi: 10.1007/s40509-015-0057-3 (see also arXiv:1508.03505)
[2] Landau, L. D., Lifshitz, E. M.: Quantum Mechanics: Non-Relativistic Theory. Volume 3, Third Edition, Elsevier Science, Oxford, 1977.
[3] Alexey Nikulov, private communication
Addendum 1:
On March 9, 2018 I receive en email from A. Nikulov containing the following statements:
В разложении бинома на слагаемые (p – qA)^2/2m = p^2/2m – pqA/m + (qA)^2/2m в книге LL принимается, что первое слагаемое описывает атом без магнитном поле, а два других в магнитном поле. Это не только нельзя ничем обосновать, но и приводит к очевидной арифметической ошибки.
Translating into English:
In the decomposition of the binom into separate terms (p – qA)^2/2m = p^2/2m – pqA/m + (qA)^2/2m in the LL book it is assumed that the first term describes the atom without the magnetic field, while two other in a magnetic field. Not only there is no way to justify it, but also it leads to an evident arithmetic error.
Again A. Nikulov is making an error. The first term is nothing else but ![]() , which is the same as
, which is the same as ![]() with
with ![]() set to zero, that is the free Hamiltonian, corresponding to the atom without the magnetic field. It is not true that Landau and Lifshitz state that two other term describe the atom in a magnetic field. They do not say so. What describes the atom in magnetic field is all three terms. All that is evident and follows from the definitions. There is nothing that need to be justified and there are no arithmetic errors.
set to zero, that is the free Hamiltonian, corresponding to the atom without the magnetic field. It is not true that Landau and Lifshitz state that two other term describe the atom in a magnetic field. They do not say so. What describes the atom in magnetic field is all three terms. All that is evident and follows from the definitions. There is nothing that need to be justified and there are no arithmetic errors.
Addendum 2:
As a reply to the Addendum 1 above, I received the following:
я не понимаю, где я опять сделал ошибку. Я согласен с тем, что The first term is nothing else but Н_0, which is the same as Н with А set to zero, that is the free Hamiltonian, corresponding to the atom without the magnetic field. Но я не могу согласится с тем, что It is not true that Landau and Lifshitz state that two other term describe the atom in a magnetic field. Даже если Landau and Lifshitz об этом ничего не написали, то что two other term describe the atom in a magnetic field очевидно из того, А в них не равно нулю. Приравняйте в выражении (8) или (10) Вашего Comment магнитное поле нулю во всех слагаемых (а не в одном!) и Вы получите выражение Н = Н_0 без арифметической ошибки (в котором кинетическая энергия равняется кинетической энергии), но и без энергии магнитного момента в магнитном поле. Мне странно объяснять, что нельзя в одном слагаемом суммы принять А = 0, а в остальных оставить ненулевое значение А. Но мне пришлось это сделать в очередном комментарии к своей статье “Could ordinary quantum mechanics be just fine for all practical purposes?” на ResearchGate. Неужели Вам непонятно, если сделать такую же глупость, какая сделана в книге Landau and Lifshitz, то мы получим арифметическую ошибку?
Below is my English translation of the relevant part, separated into two parts, intertwined with my explanations.
I do not understand when have I made again an error. I agree that “The first term is nothing else but Н_0, which is the same as Н with А set to zero, that is the free Hamiltonian, corresponding to the atom without the magnetic field.” But I cannot agree that it is not true that “Landau and Lifshitz state that two other term describe the atom in a magnetic field. Even if Landau and Lifshitz did not write it, it is clear that the two other terms describe the atom in a magnetic field, because A there is not equal zero.
My comment: Because we are discussing questions of “arithmetic errors” and “illegal substitutions” in a popular textbook, it is very important to be very precise and logically impeccable. The way it stated in the quotation above it is not logically precise and it is potentially misleading. The two terms do not constitute the full description of the atom in a magnetic field. The full description is given by all three terms together. The first term is the kinetic energy of the free particle. The second term is the linear coupling of the angular momentum to the magnetic field, the third term, quadratic in the magnetic field, is usually neglected completely for weak magnetic fields.
Perhaps it should also be stated why sometimes the talk is about the atom, while only electron figures out in the Hamiltonian. The reason is that the terms are inversely proportional to the mass, therefore the proton energy terms are small compared to the electron energy terms and can be neglected.
If you set magnetic field to zero in the expression (8) or (10) of your comment in all terms, (and not only in one!) then you will get the expression without arithmetic error (in which kinetic energy is equal to the kinetic energy), but without the energy of the magnetic moment in magnetic field. t is strange that I have to explain that you should not to set A=0 in one term, and to let it to be non-zero elsewhere.
The sentence above does not make sense. In fact ![]() has been obtained from (8) or (10) by setting the magnetic field to zero in all terms.
has been obtained from (8) or (10) by setting the magnetic field to zero in all terms.