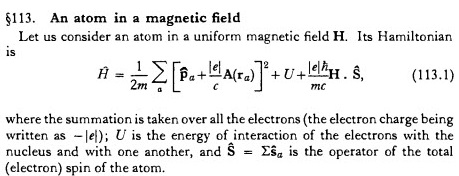
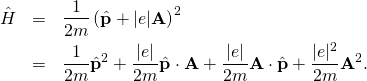In November 2015 I was reading “Quantum mechanics and gravity” by Mendel Sachs [1]. There, on p. 46 I have found the following piece that got my interest
We may exploit these ideas of generalizing Einstein’s tensor formalism by starting with the invariant differential metric in a Riemannian spacetime:
(3.10)
where
is a four-vector field (dependent on all of the coordinates
of spacetime) in which all four vector components are quaternion-valued, rather than being real-number valued. Thus the generalized metrical field
is a 16-component variable. The invariant metric
of (3.1) is a factorization of the Riemannian metric,
(3.2)
where the asterisk denotes the quaternion conjugate of
(corresponding to time reversal or space reflection).
The correspondence between the metric tensor and the quaternion metric is then, from (3.1) and (.2),
where the minus sign is chosen because of normalization.
Sachs goes then on to derive “quaternion field equations”. I got interested. After some search I have found the 1968 paper by H. G. Loos [2] criticizing Sachs’ idea of “factorizabiility of Einstein’s equations”, together with Sachs’ reply [3].
I looked and it was rather clear to me that Loos was right, nevertheless Sachs evidently persisted till 2004, and Springer did not do too well with their peer review. For some reason they were suspiciously forgiving.
Anyway I got interested and it was clear to me that Sachs used quaternions where Clifford algebras were more appropriate. Probably he did not know much about them and quaternions sounded like something more mysterious. So I decided to come back to my studies of Clifford algebras. I have used them before in my papers on conformal compactification of the Minkowski space and also in my book about Quantum Fractals. But this time I decided to study also more general Clifford algebras, namely those corresponding to possibly degenerate metrics. In fact at some point in the past I got interested in degenerate metric and wrote a paper on this subject [6] – though never published in a journal. Recently while reading “warped Passages” by Lisa Randall [7] it occured to me that Clifford algebras with degenerate metric may find new applications in multidimensional models, wher our four dimensional space time is embedded as a singular brane in a higher dimensional geometry. Sub-Riemannian geometry that I was always neglecting ay be of help here.
So I got again interested in Clifford algebras, but this time paying attention also to those features that survive when the metric becomes degenerate. In fact the exterior algebra is a special case of the Clifford algebra, therefore whoever is interested in multivectors must also be interested in Clifford algebras with degenerate metric. Physicists, till now, are either using exterior algebra, which means Clifford algebra for zero metric, or “space-time algebra” which is Clifford algebra with (nondegenerate) Minkovski metric. But what happens in between? What kind of mathematics is there?
Very soon I discovered that the subject has been discussed in the literature, mainly by Ablamowicz [8] and Ablamowicz and Lounesto [9]. It has even morphed into the discussion of “Clifford algebras of a bilinear form with an antisymmetric part” [10]. But then I noticed that these authors are quoting 1986 paper by Oziewicz [11], but they are not quoting the classic Bourbaki 1959 [12]. It appeared to me that this particular part of the Bourbaki’s “Elements of Mathematics”, dealing with sesquilinear and quadratic forms, has not been translated from French into English. If that is true, then I do not understand why? It is a beautiful exposition of the theory of quadratic forms and Clifford algebras, and evidently not always taking the same path as the treatice by Chevalley, even if Chevalley was a member of the Bourbaki group.
I started to study Bourbaki’s Algebra Chapter 9 and realized that it contains real pearls – it contains results and formulas that later on have been “discovered” and popularized among physicists by Oziewicz, Hestenes, Graf, Lounesto, Ablamowicz , and that applies as well to the case of nondegenerate quadratic forms!
At that point I decided to start writing my own “Notes on Clifford Algebras” – which is my own rewriting of what I have found in Bourbaki and elsewhere, together with step-by-step expanding the subject, adding observations that I consider as interesting.
My Notes on Clifford Algebras are available at this link.
At present, and I am writing this post on January 24, 2019, there are 22 pages, and it is release version 0.8b. Every couple of days I am adding something new or improving. Therefore I will continue this post adding “What’s new” with each version update.
References
[1] Mendel Sachs, Quantum Mechanics and Gravity, Springer 2004
[2] H. G. Loos, Factorizability of Einstein’s Field Equations, Nuovo Cimento, 55 B, 339-343, 1968
[3] M. Sachs, Comments on a letter by H. G. Loos on “Factorizability of Einstein’s Field Equations”, Lett. Nuovo Cimento, vol. 1 N 15, 741-745, 1969
[4] A. Jadczyk, On Conformal Infinity and Compactifications of the Minkowski Space, Advances in Applied Clifford Algebras, 21 N 4, 721-756, 2011
[5] A. Jadczyk, Quantum Fractals, World Scientific 2014
[6] A. Jadczyk, Vanishing Vierbein in Gauge Theories of Gravitation, arXiv:gr-qc/9909060v1
[7] Lisa Randall, Warped Passages, HarperCollins 2006
[8] Rafal Ablamowicz, Structure of spin groups associated with degenerate Clifford algebras, Journal of Mathematical Physics, 27, 1-6, 1986
[9] Rafal Ablamowicz and Pertti Lounesto, Primitive Idempotents and Indecomposable Left Ideals in Degenerate Clifford Algebras, in J. S. R. Chisholm and A. K. Common ed, Clifford Algebras and Their Applications in Mathematical Physics, Reidel 1986
[10] Rafal Ablamowicz and Pertti Lounesto, On Clifford Algebras of a Bilinear Form with an Antisymmetric Part, in R. Ablamowicz and J. M. Parra and P. Lounesto ed., Clifford Algebras with Numeric and Symbolic Computations, Birkhäuser 1966
[11] Zbigniew Oziewicz, From Grassmann to Clifford, in Clifford Algebras and Their Applications in Mathematical Physics, 1986, op. cited
[12] N. Bourbaki, Algèbre – Chapitre 9. Hermann, 1959.
[13] Claude Chevalley, The Algebraic Theory of Spinors and Clifford Algebras: Collected Works, Volume 2, Springer 1996.
What’s new v0.8c, 26/1/2019
Added 1.1.5 Diagonalization of symmetric bilinear forms
Added Even and odd subalgebras at the end of 1.2.2 Main involution and main anti-involution