April 2000:  THE THIRD EXPEDITION

The ship came down from space. It came from the stars and the black velocities, and the shining movements, and the silent gulfs of space.
….
“Mars!” cried Navigator Lustig.
“Good old Mars!” said Samuel Hinkston, archaeologist.
“Well,” said Captain John Black.
The rocket landed on a lawn of green grass.
….
“I’ll be damned,” whispered Lustig, rubbing his face with his numb fingers. “I’ll be damned.”
“It just can’t be,” said Samuel Hinkston.

Ray Bradbury, The Martian Chroniques

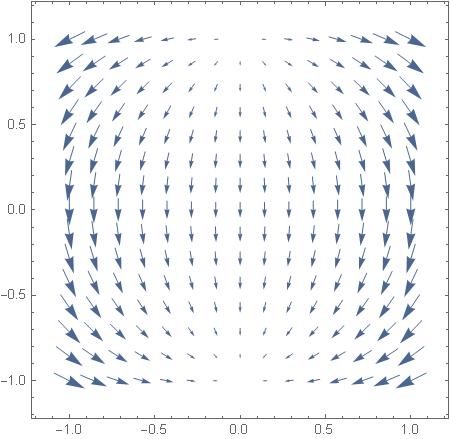
And we are here on our third field expedition. During the first expedition we had seen the stream moving down:

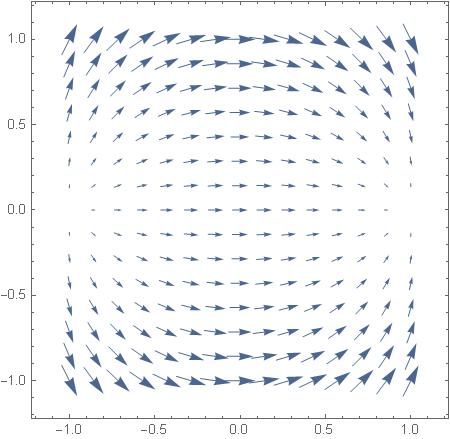
Then, in our second expedition the stream was flowing to the right.

What will happen in our third expedition? Will it flow up now? No. It will be something else. You will say “I’ll be damned, It just can’t be.” And yet here it is:

This time we take

   

The vector field comes as

   

Its plot is:

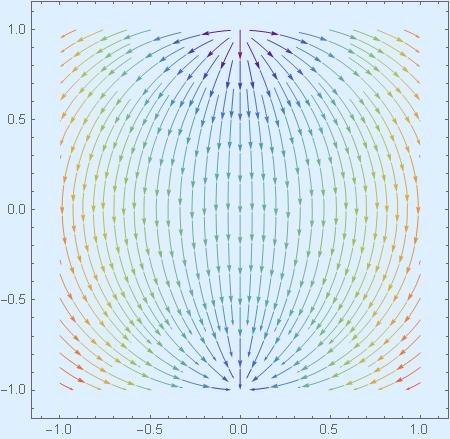
And the streamlines:

Oh, no, sorry, these are from Mars. Mathematica gives these:

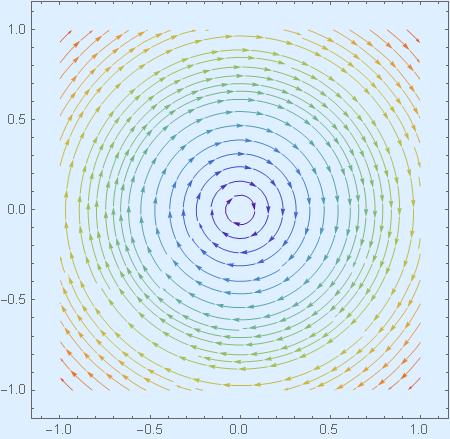
Now the mystery is this: Combining 1 and 2 one can get 3, combining 2 and 3 one can get 1, combining 3 and 1 one can get 2. Perhaps. It resonates, doesn’t it?

But how exactly this happens? Where are the details that devil dwells in?

Our first field expedition was not without adventures. What kind of adventures they were – this can be now only guessed looking at some cryptic comments. They refer to the text that does not exist any more. I have changed the example. Last night Goddess talked to me in my dream, and She suggested a different avenue from that that I have had in my mind before. I obey. And so the second example follows. Very similar to the previous one, just a little been different.

Last time I considered the one parameter group of transformations defined, on the complex plane, by

   

where

Today I propose a variation:

   

Again, with some little effort (perhaps easier than it was before, because now there is no more imaginary explicitly in the formula) we can verify that

and

In order to obtain the vector field from this “flow of complex numbers” I am calculating the derivative:

   

I used Mathematica to get

   

To draw this vector field on the plane I write and calculate

   

So

   

Here is the vector field, and stream lines from this second field expedition

Of course there will also the third expedition. After that the mystery will slowly be be revealed.

Whenever there is a path, there are vectors tangent to that path. Like here, on the picture below. I went outside for a walk, in search of vector fields. At first couldn’t find any, but then I realized that there is a vector tangent to my path, at every point of my path. Here are three such vectors:

But then, I looked to the right of the path, and there I saw a real, two-dimensional field of vectors.

Then, at home, I used Mathematica to produce an artificial vector field:

And I have generated its stream lines:

To every vector field we have associated flow represented by steam lines, and to every flow of stream lines we have associated vector field. We generate stream lines from vector field by integration, we generate vector filed from flow of stream lines through differentiation.

Here is how I did it in order to create the two graphs above:

I thought about one-parameter family of transformations on the complex plane, but not too trivial one. Something that I did not know in advance how exactly it would look like. The following has occurred to me:

   

where Notice that and To check the first property takes a little bit of calculations. In order to obtain the vector field from this “flow of complex numbers” I am calculating the derivative:

   

I used Mathematica to get

   

To draw this vector field on the plane I write and calculate

   

So