Category: Uncategorized

Luminous eggs

Posted on by arkajad

“An incomplete person has a hole in the stomach,” she went on. “A sorcerer can see it as plainly as you can see my head. When the hole is on the left side of one’s stomach, the child who created that hole is of the same sex. If it is on the right side, the child is of the opposite sex. The hole on the left side is black, the one on the right is dark brown.”

“Can you see that hole in anyone who has had children?”

“Sure. There are two ways of seeing it. A sorcerer may see it in dreaming or by looking directly at a person. A sorcerer who sees has no problems in viewing the luminous being to find out if there is a hole in the luminosity of the body. But even if the sorcerer doesn’t know how to see, he can look and actually distinguish the darkness of the hole through the clothing.”
…..

“It’s very hard to tell,” she said after considerable coaxing. “She is the same as you and me, and yet she’s different. She has the same luminosity, but she’s not together with us. She goes in the opposite direction. Right now she’s more like you. Both of you have patches that look like lead. Mine is gone and I’m again a complete, luminous egg.

Carlos Castaneda, The second ring of power.

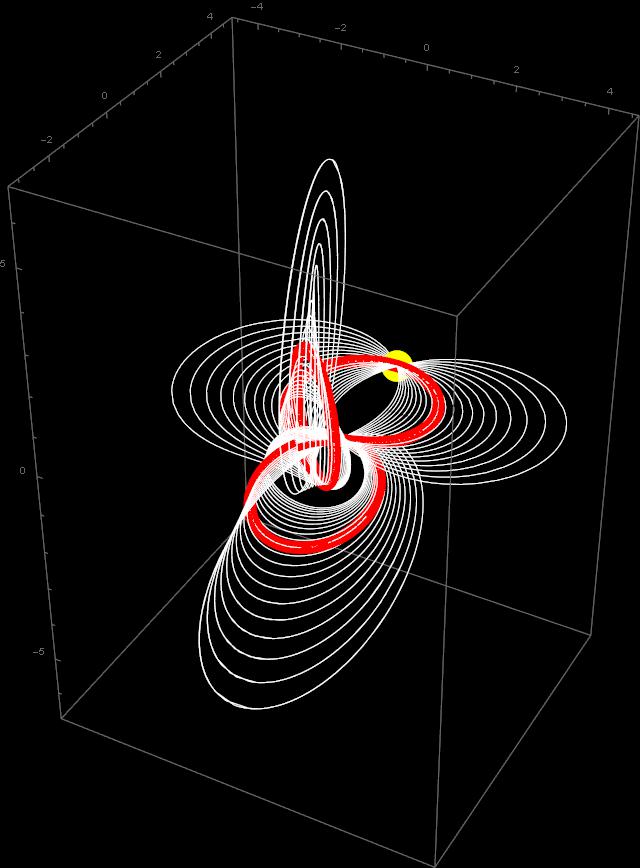
Today I was playing with trajectories for different values of d.
Below are trajectories for d=0.6, d=0.7 and d=0.8.
I was adjusting time (between 100 and 1000, and angle of view, so as to show
the interesting side of the figure.

Of course I could also rotate in dour dimensions. This would change the shape in 3D in a strange way.
So, here are the pictures:

d=0.6

d=0.7

d=0.8

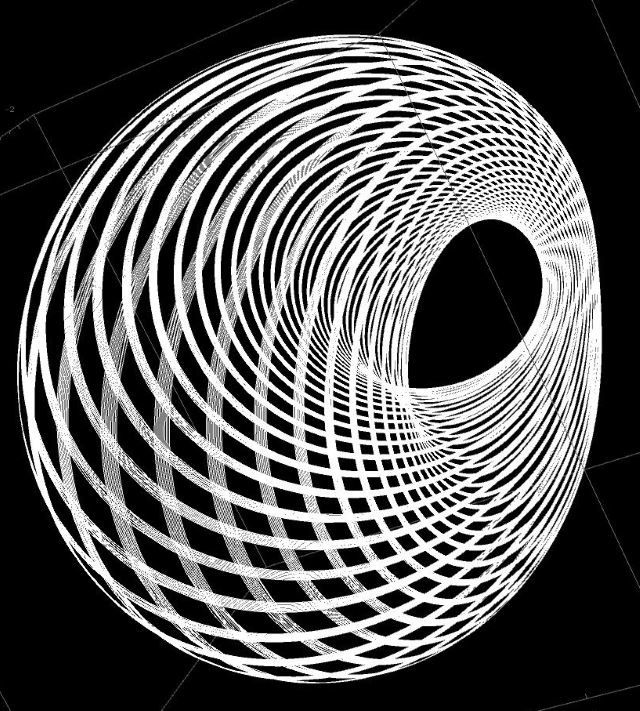
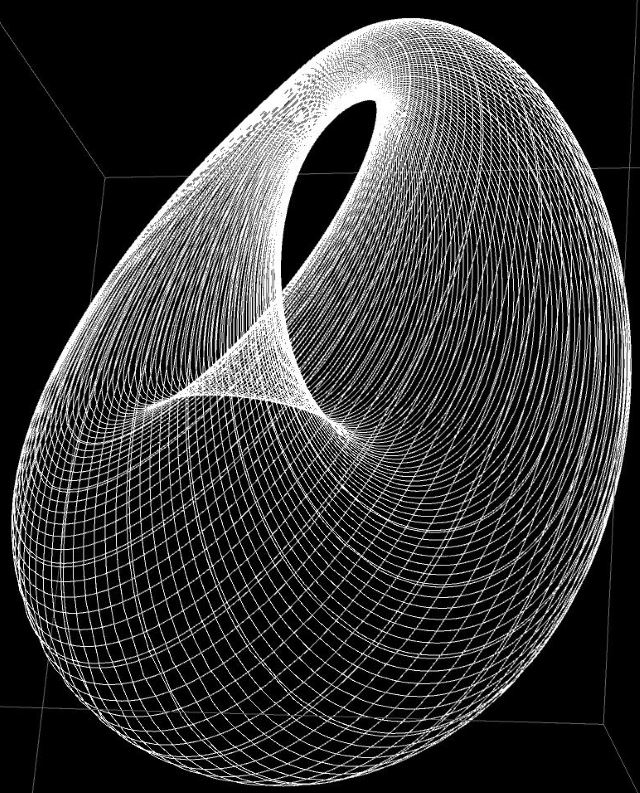
Making these pictures is somewhat time consuming. Even with compiled functions the computer has to calculate Jacobi elliptic functions am, sd and pi for each point. And to make one trajectory I use 10 000 points, otherwise it may show bad zig-zags.

Probably I could do it faster writing a Fortran program, but writing such a program would take even more time.

Zero in disguise

Posted on by arkajad

You look like an angel
Walk like an angel
Talk like an angel
But I got wise
You’re the zero in disguise
Oh, yes, you are
The zero in disguise

Elvis was singing about you ….

Ronan, in his recent comment under my post on Quaternion evolution, has noticed that Maple, the software he is using, gives for the function \psi(t) result that than the one I was advocating. How can it be? Mathematics is an exact science (physics, on the contrary, is not exactly an exact science). For a given question there should be one answer. Or is mathematics a devil in disguise? Looks like an exact science, walks like an exact science, but exact science it is not?

Devil likes to hide in the details, so let us examine the details.

My formula for \psi(t) comes from integration. I claim that if we integrate

(1)   \begin{equation*} \psi(t)=\int_0^t\frac{\cosh( \frac{s}{2\sqrt{3}})+1}{2\cosh(\frac{s}{2\sqrt{3}})+1}\,ds \end{equation*}

the result is given by rather simple a formula:

(2)   \begin{equation*} \psi_A(t)=\frac{t}{2}+ 2\arctan\left(\frac{\tanh \left(\frac{t}{4 \sqrt{3}}\right)}{\sqrt{3}}\right). \end{equation*}

Ronan, who is using Maple, gets for the same problem a different answer:

(3)   \begin{multline*} \psi_R(t)=\pi i \sqrt{3}+2\arctan(\frac{1}{3}\tanh(\frac{1}{12}t\sqrt{3})\sqrt{3})\\-\sqrt{3}\ln\left(\tanh\left(\frac{1}{12}t\sqrt{3}\right)-1\right)+\\\sqrt{3}\ln\left(\tanh\left(\frac{1}{12}t\sqrt{3}\right)+1\right). \end{multline*}

Taking the difference we get

(4)   \begin{multline*} \psi_A-\psi_R=\frac{t}{2}-\pi i\sqrt{3}+\\\sqrt{3}\ln\left(\tanh\left(\frac{1}{12}t\sqrt{3}\right)-1\right)-\sqrt{3}\ln\left(\tanh\left(\frac{1}{12}t\sqrt{3}\right)+1\right). \end{multline*}

If mathematics is not a devil in disguise, the last expression, namely \psi_A-\psi_R must be a zero in disguise. But is it?
I tried to ask Maple to simplify it, with no result. The algorithms known to otherwise very clever software are not smart enough. So, let is see what we can do with our eyes and hands.

The first thing that comes to our attention is that we have a difference of logarithms. And we know from the kindergarten that \ln a-\ln b=\ln\frac{a}{b}. So we write

(5)   \begin{equation*} \psi_A-\psi_R=\frac{t}{2}-\pi i\sqrt{3}+\sqrt{3}\ln\frac{\tanh\left(\frac{1}{12}t\sqrt{3}\right)-1}{\tanh\left(\frac{1}{12}t\sqrt{3}\right)+1}. \end{equation*}

But what is \tanh? We know that \tanh x=\sinh x/\cosh x, therefore

(6)   \begin{equation*} \frac{\tanh(x)-1}{\tanh(x)+1}=\frac{\frac{\sinh x}{\cosh x}-1}{\frac{\sinh x}{\cosh x}+1}=\frac{\sinh x -\cosh x}{\sinh x+\cosh x}. \end{equation*}

Now, \cosh x=(e^x+e^{-x})/2,\quad \sinh x=(e^x-e^{-x})/2, therefore \sinh x+\cosh x=e^x, \sinh x-\cosh x=-e^{-x}. Thus

    \[\frac{\tanh(x)-1}{\tanh(x)+1}=\frac{e^{-x}}{e^{x}}=-e^{-2x},\]

and so

    \[ \ln \frac{\tanh(x)+1}{\tanh(x)-1}=\ln -e^{-2x}.\]

We can write now

    \[\ln -e^{-2x}=\ln ((-1)e^{-2x})=\ln(-1)+\ln e^{-2x}=\ln(-1)-2x.\]

What is \ln(-1)? It is an imaginary number. We know that e^{\pi i}=-1, and if we ask Maple what is \ln(-1)? – the answer is i\pi. So, we finally get

    \[ \ln \frac{\tanh(x)+1}{\tanh(x)-1}= i\pi -2x.\]

In our case x=\frac{1}{12}t\sqrt{3}, 2x=\frac{1}{6}t\sqrt{3}.
In Eq. (5) we have \sqrt{3} in front of the \ln, so we get

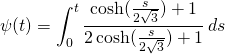
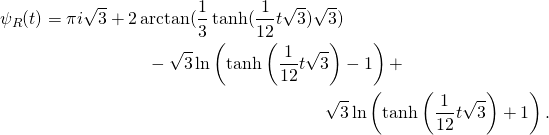
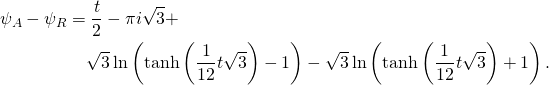
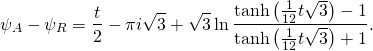
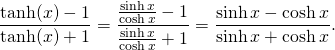
    \[\sqrt{3}\ln\frac{\tanh\left(\frac{1}{12}t\sqrt{3}\right)-1}{\tanh\left(\frac{1}{12}t\sqrt{3}\right)+1}=-\frac{t}{2}+\sqrt{3}i\pi. \]

Thus \psi_A-\psi_r=0. The zero has been cleverly disguised using functions \tanh and \ln. Einstein once commented that God is subtle, but He is not malicious. Well, here we could see Mathematics can be subtle

Being a 4D butterfly – how does it feel?

Posted on by arkajad

It was yesterday that I started writing about the idea of more than one path. I imagine being a rigid body in zero gravity. I mean not like a dead human body floating in cosmic space, rather like the T-handle experiencing Dzhanibekov effect. I am floating in space.

How does it feel being a T-handle? My space is the three-dimensional rotation group. Perhaps the group of unit quaternions, the sphere S^3. My perception, perhaps, projects it stereographically on 3-dimensional space, so it is much like for me, the human being thinking about being the T-handle. But, according to the analysis from yesterday’s post More than one path, my sky has two sets of privileged directions. If I start moving into such a privileged direction, very soon I will be close to performing eternal returns moving along essentially always the same circle.

But will it be the same circle for each of these special lines? Or they will be different circles? How do they look? These special trajectories? I am trying to figure it all out, and today I am reporting on my progress. It is all preliminary, there may be errors, perhaps my reasoning has mistakes? With time, and with help of my readers, I am sure we will get to the truth. So, here is what I think, and what I did because of my thinking.

Yesterday I was analyzing paths through the point -1. But, after some thinking and experimenting I decided that I do not like this point. We already have a very nice path, described by nice formulas, for instance in Meeting with remarkable circles

The path q(t) in unit quaternions at t=0 passes through the point q(0)=(\sqrt{3}/2,0,1/2,0). This point is in the middle between the two circles of eternal return. There is nothing wrong with this point. Therefore I want to consider all special paths that have the property that at t=0 they pass through this point. This is my task.
So, I went to work, and now I will show you the result. Which may be right, or may be wrong – I do not know yet. First pictures, then explanation how I got them:

The yellow point is the common starting point q(0)=(\sqrt{3}/2,0,1/2,0). The red path is the same as in the picture before, the path that we already know. The other paths are also special, starting at the same point, but in different special directions. There are only 21 of them – to start with. What we are getting is something similar to a butterfly. It would not, probably, be able to fly in 3D, but it may be able to fly in 4D. If you click on the butterfly image animation will open showing it from different angles, so that you can get a better idea about its space structure.

Perhaps our souls, when flying, look like that?

How did I get it? I decided that other special paths I will get choosing other solutions of the same Euler’s equations. To choose a different solution I shifted time in the solution for \mathbf{L}(t) (I did not play with signs yet). This way I get a different solution of the attitude equation. But this new solution has a different starting point. So I multiply by a constant quaternion from the left to move the starting point to q(0) above.
I did not shift time too much, just \pm 4 with spacing 0.4, so I got 21 trajectories.
Now I have to check all that and, if necessary, fix what needs to be fixed.
When all is fixed, I will share the code.