Day: April 16, 2017

Cayley transform for Easter

Posted on by arkajad

It is Easter Sunday when I writing this note. In Getting real we met the Cayley transform and its inverse.

Namely we have defined the unitary matrix \mathcal{C}

(1)   \begin{equation*}\mathcal{C}=\frac{1}{1+i}\begin{bmatrix}i&1\\-i&1\end{bmatrix}.\end{equation*}

Its inverse \mathcal{C}^{-1}=\mathcal{C}^* being given by

(2)   \begin{equation*}\mathcal{C}^{-1}=\frac{1}{1-i}\begin{bmatrix}-i&i\\1&1\end{bmatrix}.\end{equation*}

We will use the matrix \mathcal{C} in two ways: either for implementing a similarity transformation A\mapsto A'=\mathcal{C}^{-1}A\mathcal{C} resp. A'\mapsto \mathcal{C}A'\mathcal{C}^{-1}, or for implementing fractional linear transformation of the type

(3)   \begin{equation*}z\mapsto A\cdot{z}=\begin{bmatrix}\lambda&\mu\\ \nu&\rho\end{bmatrix}\cdot z= \frac{\rho z+\nu}{\mu z+\lambda}.\end{equation*}

In each case a factor, such as \frac{1}{1+i}, in front of the matrix is not important. It will cancel out. Two proportional matrices implement the same similarity transformation and the same fractional linear transformation. We have chosen the factor so as to have \mathcal{C} unitary of determinant 1, but that fact will play no role. What is important is the internal structure of \mathcal{C}.

In Getting real we have found that the similarity transformation

    \[ A\mapsto A'=\mathcal{C}^{-1}A\mathcal{C}\]

transforms complex SU(1,1) matrices into real SL(2,R) matrices. Let us now check the fractional linear transformation implemented by \mathcal{C}^{-1}. For a general case it is convenient to denote the fractional linear transformation (3) as A\cdot z. From Eq. (3) it can be easily verified that, whenever the results are finite, we have

(4)   \begin{equation*} B\cdot(A\cdot z) =(BA)\cdot z.\end{equation*}

One could extend the domain and the range of the transformation by replacing the complex plane by its one-point compactification, the Riemann sphere, but we will not need such an extension.

For us the crucial observation is that the transformation z\mapsto \mathcal{C}^{-1}\cdot z maps the unit disk D onto the upper half-plane \mathbb{H}, that is onto the set of complex numbers with positive imaginary part. To see this let us examine the properties of z' defined by

(5)   \begin{equation*}z'=\mathcal{C}^{-1}\cdot z=\frac{z+1}{iz-i}=\frac{(z+1)(-i\bar{z}+i)}{|z-1|^2}=\frac{i(1-|z|^2)+i(z-\bar{z})}{|z-1|^2}.\end{equation*}

The imaginary part of z' is evidently positive when |z|^2<1. It becomes zero when |z|^2=1. Conversely, the transformation z'\mapsto z=\mathcal{C}\cdot z'=\frac{z'-i}{z'+i} maps the upper half-plane \mathbb{H} in D. To see that this is indeed the case let us calculate |z|^2:

(6)   \begin{equation*}|z|^2=\frac{|z'-i|^2}{|z'+i|^2}=\frac{(z'-i)(\bar{z'}+i)}{(z'+i)(\bar{z'}-i)}=\frac{|z'|^2+1+i(z'-\bar{z'})}{|z'|^2+1-i(z'-\bar{z'})}.\end{equation*}

Now z'-\bar{z}'=2i\mathrm{Im}(z'), therefore if \mathrm{Im}(z')>0, then

(7)   \begin{equation*}|z|^2=\frac{|z'|^2+1-2\mathrm{Im}(z')}{|z'|^2+1+2\mathrm{Im}(z')}<1.\end{equation*}

Moreover, if \mathrm{Im}(z')=0, that is if z' is on the real axis, then its image z is on the unit circle.

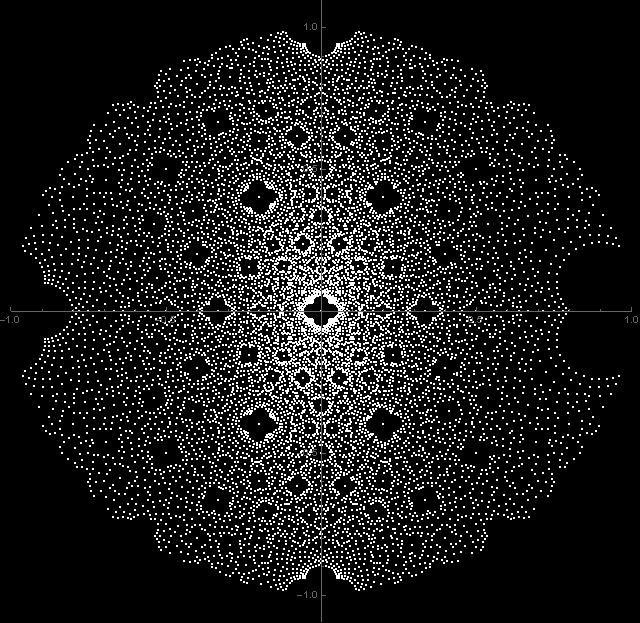
We finish this Eater post with an ornament. Consider complex numbers z' of the form z'=(m+in)/(p+iq) where m,n,p,q are real integers with \mathrm{Im}(z')>0 and pq\neq0. Apply the Cayley transform to each such number and plot the number \matcal{C}\cdot z' as a point in the disk. Of course we have to restrict the size of m,n,p,q, say to \leq 9. The result is the following Easter Ornament:

Gaussian fractions on the disk.