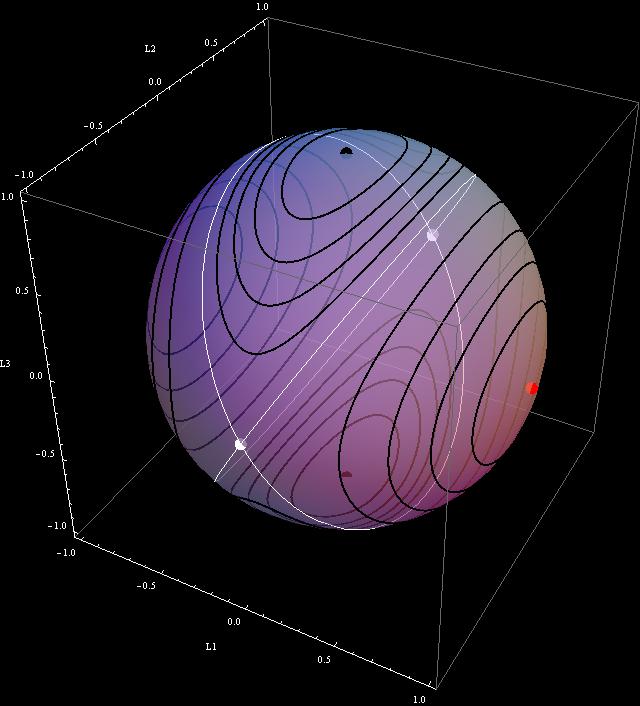
In the last post, Racket about tennis racket, I did not quite finish with the critical appraisal of the Poinsot-type picture:
According to wikiHow to Critique Artwork I should really do the following:
2
Analyze the artwork. Evolve the art criticism from a technical description to an in-depth examination of how the technical elements were utilized by the artist to create the overall impression conveyed by the artwork. Technical elements you need to analyze when you critique artwork include:Color.
Shapes, forms and lines.
Texture.
Light and shadow.
How each technical element contributes to the mood, meaning and aesthetic sensation of the artwork.
But even before doing that, there is something very very very important that was in part 1: “Objects in the painting” – that I did not delve deep enough into. The point is that the artist is presenting here certain particular impressions about a certain object. And the object is not some abstract sphere in some “angular momentum space”. The object is real, is pretty, is simple, and is mysterious – in our ordinary 3D space, floating over the water, under blue cloudy skies. Here it is:
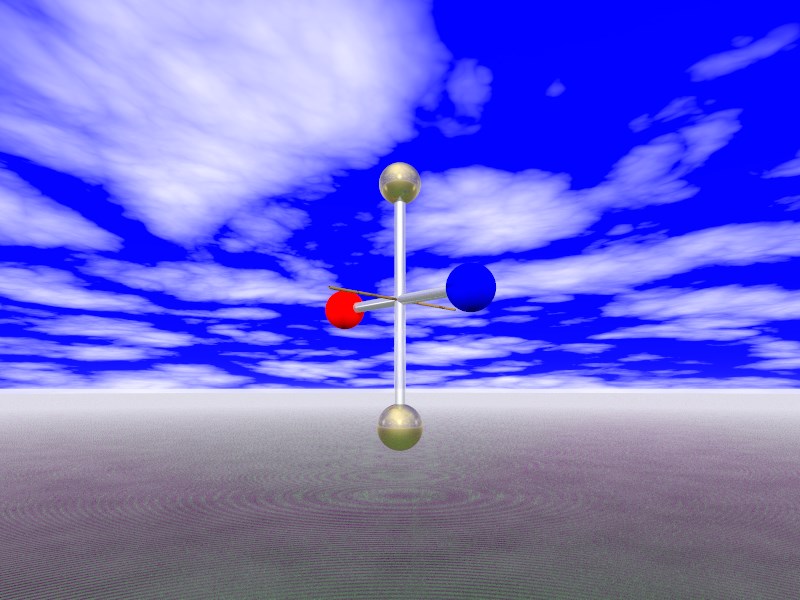
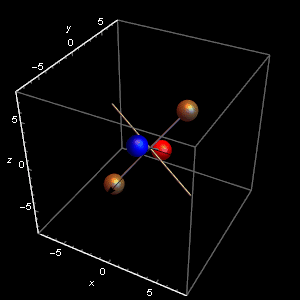
The Top object
So, what is this object? It is not a drone. More like an UFO. It consists of four spherical masses. It is not important how large these spheres are. Here they seem to be quite large, but, in fact, life is simpler when they shrink to points. What is important is that they are heavy. Each mass in the picture is, say, 1/2 kg. The blue and red masses are connected by a weightless rod of length 2m. The bronze metal masses are connected by a weightless rod that is ![]() m long. There is also the third, thin and weightless, rod that is perpendicular to the other two. It plays no role whatsoever, no masses are attached to its ends. It is there just for pure pleasure of the Creator.
m long. There is also the third, thin and weightless, rod that is perpendicular to the other two. It plays no role whatsoever, no masses are attached to its ends. It is there just for pure pleasure of the Creator.
The center of mass of our top is at its geometrical center, where the joining rods intersect. Let us agree that the axis joining the bronze masses is the first, say, ![]() -axis. The axis joining red and blue masses is the second,
-axis. The axis joining red and blue masses is the second, ![]() -axis, and the perpendicular axis, with no masses attached, is the third, the
-axis, and the perpendicular axis, with no masses attached, is the third, the ![]() -axis.
-axis.
Let’s recall how we calculate the moments of inertia of a system of masses. Here is the extract from Hyperphysics site:
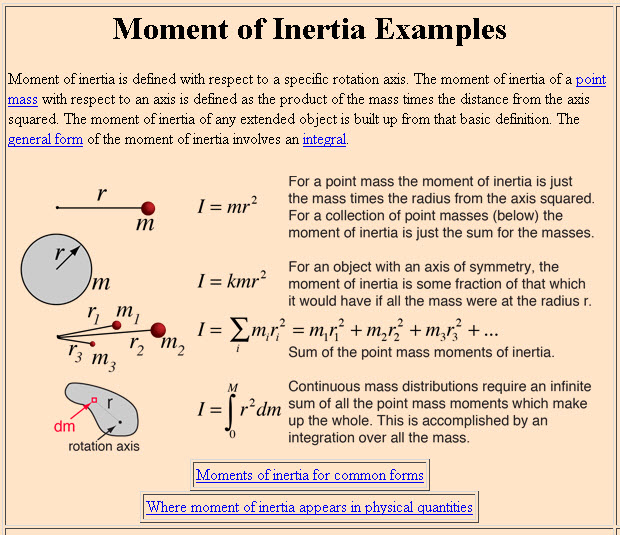
We calculate now the moment of inertia ![]() with respect to the first axis. There are two masses, each
with respect to the first axis. There are two masses, each ![]() , rotating about this axis, each at the distance
, rotating about this axis, each at the distance ![]() . The moment of inertia (I will skip the units, like kg and m) is
. The moment of inertia (I will skip the units, like kg and m) is
![]()
Now we calculate ![]()
![]()
And ![]()
![]()
Now let us see these moments of inertia in a spectacular action of freely rotating in space. Not just rotating! Rotating and the flipping.
Click on the image to open the animation in a new window. Depending on your connection it may take a while. The size is almost 1 MB. You will see the flips. But why? What causes these flips?
Yes, these balls do move and flip! As if they were alive! Yes, rotating and spinning and flipping opens the door of perception to other dimensions. And we will learn it all. No joking, it all. But now we have a movie, and we will have to learn about How to Write a Movie Review…