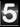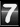In the last note, Towards the road less traveled with spin, we were looking at the asymmetric top spinning about its z-axis, and we have analyzed the path taken by the top, using the stereographic projection. The result turned out to be really boring. A straight half-line, not even worse of looking at. But who or what is the guilty party? The object of the painting, the spinning top, or the painter?
On the net you can find 3 Handy Tips For Learning to See Like an Artist. The second of these tips concerns us:
2. Turn Your Subject Upside Down
We tend to have the most difficulty drawing what we see when we are drawing things we are very familiar with, like facial features, body parts etc.
Because we know we are drawing an eye, for example, instead of relying on what we are seeing, our brain takes over and starts saying “this is what an eye should look like”.
But if we turn the reference (and our drawing) upside down, the features appear much less familiar, and we essentially trick our brain into staying quiet so that we can get on with drawing what we see.
Obviously if you’re drawing from life, you can’t turn your model upside down, but even just turning your drawing around in 90 degree steps can help you see areas where your drawing isn’t quite accurate. You can tilt your head 90 degrees too, to get a fresh look at the model.
And that is what we are going to. But why 90 degrees? We will first try 30, the 60 degrees and see what comes out? And we will also turn around, for even better experience.
You will see that the road becomes more interesting, we will experience something similar to the passengers of the Serra Verde Express

Here are the details.
Suppose we tilt our head 30 degrees left. The effect is the same as if the whole universe, including our spinning top, would tilt right. It is rather difficult to tilt the whole universe without disturbing it. But we can do it in our mind. We do not want to touch the top while it spins. If we do, it will change its rotation, probably it would start to nutate. Like on this movie at 1:18
If we want to have our top rotating about its z-axis, but tilted, we should first tilt it, and only then set in motion respecting its orientation. It is safer to tilt our head or, more scientifically, to tilt the laboratory frame.
Suppose we want to tilt the laboratory frame by 30 degrees about its t-axis. In Towards the road less traveled with spin) we have found that rotation about the z-axis is described by the matrix
(1) ![]()
Using the same method we can find that rotation by the angle ![]() about y-axis is described by the matrix
about y-axis is described by the matrix
(2) 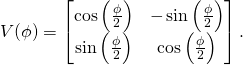
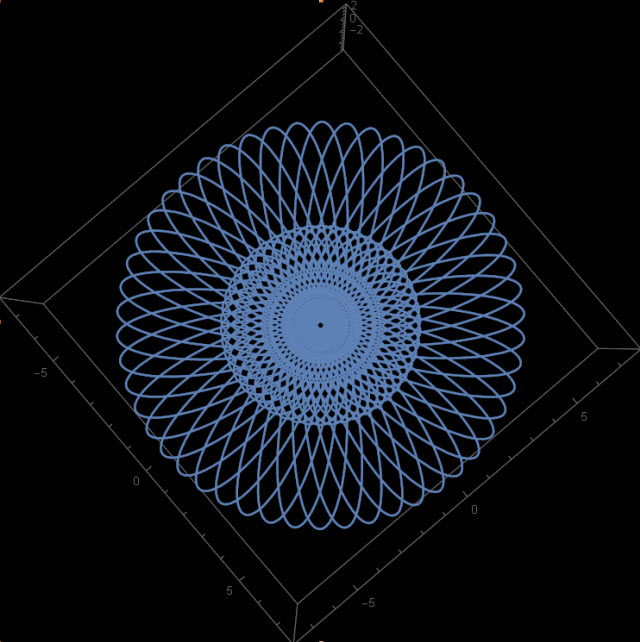
Rotation of the laboratory frame is described by acting from the left. Therefore rotation of the top observed from the tilted reference frame is described by the product ![]() That way we will obtain a trajectory. The axes of the tilted top will never coincide with the axes of the laboratory frame. Therefore the stereographically projected trajectory will never escape to infinity. We can plot it all. But one trajectory would be boring. It is better to tilt the laboratory frame (all the time by 30 degrees) using 50 different tilting axes (all in the xy plane. The following picture is then produced:
That way we will obtain a trajectory. The axes of the tilted top will never coincide with the axes of the laboratory frame. Therefore the stereographically projected trajectory will never escape to infinity. We can plot it all. But one trajectory would be boring. It is better to tilt the laboratory frame (all the time by 30 degrees) using 50 different tilting axes (all in the xy plane. The following picture is then produced:
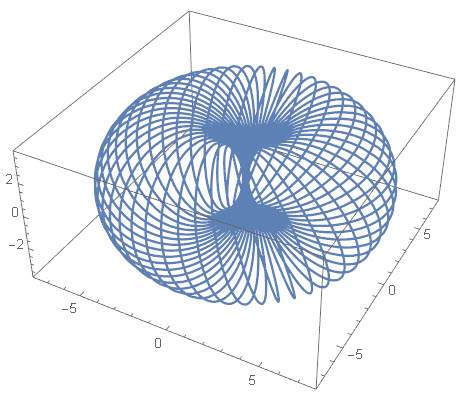
If we add trajectories from 60 degree tilts and look from the top, we get something that is not much worse than Serra Verde Express:
We can also try this tip: By simply squinting your eyes as you look at the subject, you can eliminate a lot of the detail, making it much easier to see simple shapes and values.
In the following posts we will be squinting our top.