Left circular orbits in SU(1,1)

Posted on by arkajad

We continue, from the previous post, with the beauty of the group SU(1,1). We already know that it looks like a doughnut, it feels like a doughnut, and it tastes like a doughnut. Yummy.

We represented SU(1,1) as a product of the open unit disk D and the circle parametrizing it by z and \theta. The circle group U(1) can be considered as a subgroup of SU(1,1). We analyzed orbits of the right action of U(1) on SU(1,1) using the (z,\theta) parametrization:

The orbits are circles going around the doughnut. Every point of D determines one orbit.

What about orbits of the left action? The truth is: we will not need them in what follows, but it is natural question to ask, so why not to ask?

So, let us take U(t)\in \mathrm{U}(1) of the form

(1)   \begin{equation*}U(t)=\begin{bmatrix}e^{it}&0\\0&e^{-it}.\end{bmatrix}\end{equation*}

We take A in SU(1,1), represent it as A=P(z)U(\theta), and we contemplate the product U(t)A – with A fixed and t running from 0 to 2\pi, we get one orbit. In order to represent the points of this orbit in terms of parameters z and \theta, we need to represent U(t)A as P(z')U(\theta'). We do it by using a little trick, one of the standard tricks that every magician knows. We write

(2)   \begin{equation*} U(t)A=U(t)P(z)U(\theta)=U(t)P(z)U(t)^*U(t)U(\theta)=U(t)P(z)U(t)^*U(\theta+t).\end{equation*}

Here we used the fact that U(t) are unitary, U(t)U(t)^*=I, and that they have the group property U(t)U(\theta)=U(\theta+t). From the fact that U(t) are unitary follows even more. P(z) is Hermitian, therefore U(t)P(z)U(t)^* is also Hermitian. Moreover, U(t)P(z)U(t)^* has the same eigenvalues as P(z). Since P(z) is positive, therefore U(t)P(z)U(t)^* is also positive, and it is also an an element of SU(1,1), therefore it must be of the form P(z') for some z'. By direct calculation we find that

(3)   \begin{equation*}U(t)P(z)U(t)^*=\begin{bmatrix}e^{it}&0\\0&e^{-it}\end{bmatrix}\begin{bmatrix}\frac{1}{\sqrt{1-|z|^2}}&\frac{\bar{z}}{\sqrt{1-|z|^2}}\\ \frac{z}{\sqrt{1-|z|^2}}&\frac{1}{\sqrt{1-|z|^2}}\end{bmatrix}\begin{bmatrix}e^{-it}&0\\0&e^{it}\end{bmatrix}=\end{equation*}

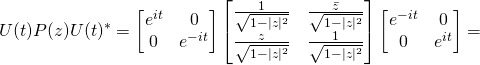
    \[ =\begin{bmatrix}\frac{1}{\sqrt{1-|z|^2}}&\frac{e^{2it}\bar{z}}{\sqrt{1-|z|^2}}\\ \frac{e^{-2it}z}{\sqrt{1-|z|^2}}&\frac{1}{\sqrt{1-|z|^2}}.\end{bmatrix}\]

Now, since |e^{-2it}z|=|z|, we deduce that z'=e^{-2it}z. Therefore the orbit is a path around the donut that, while going around, moves on a circle around the center of the disk – the cross-section of the donut. While going once around the donut, it makes two full circles in the disk – a closed spiral.

The centers of the disks form a separate orbit. It is the orbit of both right and left action. The centers of the disks correspond to z=0.

Left circular orbits. The apparent irregularity on the left is the effect of a perspective and a particular angle of viewing.

So, we know the orbits of U(1) acting from the left. As I mentioned above, we will not need them. What we will need is the description of the left action of the group S(1,1) on the space of right circular orbits. This will be explained in the next post, and the kitty is already waiting

7 thoughts on “Left circular orbits in SU(1,1)”

  2. Ark,
    there is a question:
    if you chose something why did you choose it?

    Why your doughnut is not a horn torus?
    In other words:
    why the distance from center of torus to center of disk is (nearly?) 2 in your picture?

    1. “why the distance from center of torus to center of disk is (nearly?) 2 in your picture?”

      Good question. In this case it is like choosing a color of your shirt for a given day. Hard to justify your choice, but some choice is necessary.

      I want to take the unit disk and rotate it to make a torus-like shape. The radius of rotation is inessential, it is a question of esthetics and convenience.

      1. Anyway some radiuses may be outstanding.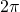
        For example radius equal to 1 would make the length of perimeter of centers disks circle equal to 2\pi.

      2. “I want to take the unit disk and rotate it to make a torus-like shape.”

        Horn torus has a torus-like shape (although a specific/distinctive/outstanding one).

        1. I admit, I didn’t know what is horn torus. Now I know. The disadvantage of using it is that it may suggest that two points on opposite sides but very close to the central axis are very close in the group. Which is not the case.

Leave a Reply